“Scientists and philosophers… had always assumed that the world worked by physical laws, and if you could measure initial conditions accurately enough, those laws would let you predict the future indefinitely. As James Gleick described it in his book Chaos: Making a New Science, this view was very wrong.”
“There was always one small compromise, so small that working scientists usually forgot it was there, lurking in a corner of their philosophies like an unpaid bill. Measurements could never be perfect,” he wrote. “Scientists marching under Newton’s banner actually waved another flag that said something like this: Given an approximate knowledge of a system’s initial conditions and an understanding of natural law, one can calculate the approximate behaviour of the system. This assumption lay at the philosophical heart of science.”
“Today we know how wrong this assumption was. The Three Body Problem is now recognized as a classic example of a chaotic system. Like the butterfly that causes a hurricane by flapping its wings, it is exquisitely sensitive to initial conditions. The tiniest tweak can have massive consequences down the line.”
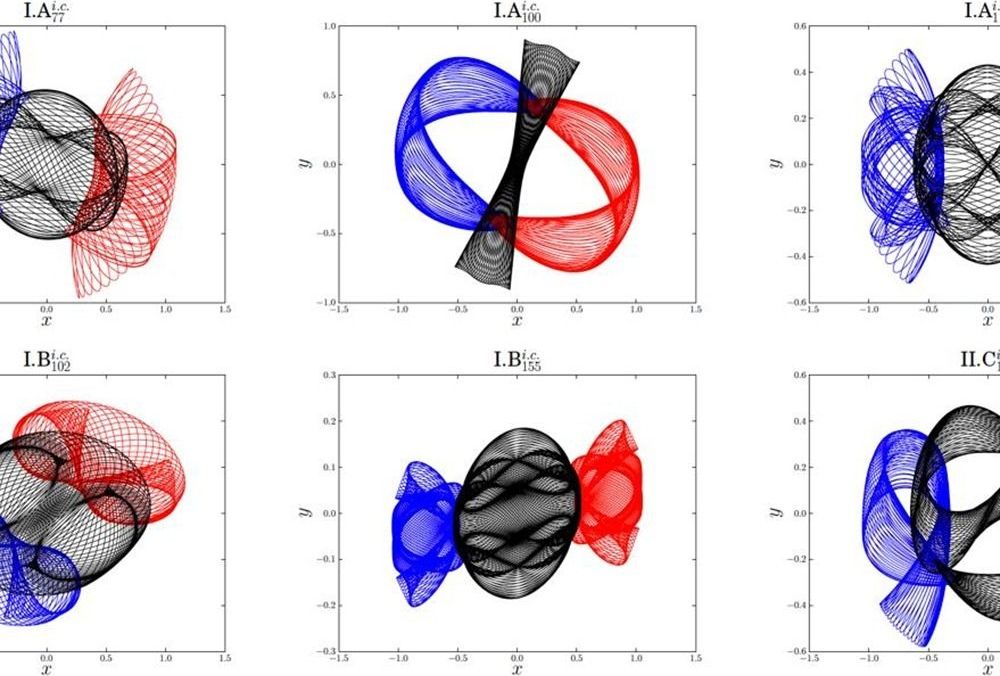
Like the endlessly repeating patterns of chaos theory, the new solutions discovered by the Chinese researchers make for elaborate and weirdly beautiful images when they are plotted in two dimensions. They are unlikely to have ever existed in reality, however. Because of how solar systems form, planets, moons and stars tend to settle into regular orbits on a single plane.
The Chinese researchers credit their discoveries to advancements in computer science and their novel technique called clean numerical simulation, which is a strategy for modelling chaotic systems, in which solutions are reached indirectly by continuous refinement, rather than directly by brute calculation.
“In this paper, we numerically obtain 695 families of Newtonian periodic planar collisionless orbits of three-body system with equal mass and zero angular momentum in case of initial conditions with isosceles collinear configuration, including the well-known figure-eight family found by Moore in 1993, the 11 families found by Šuvakov and Dmitrašinović in 2013, and more than 600 new families that have never been reported, to the best of our knowledge,” reads the report by XiaoMing Li and ShiJun Liao.








Comments are closed.